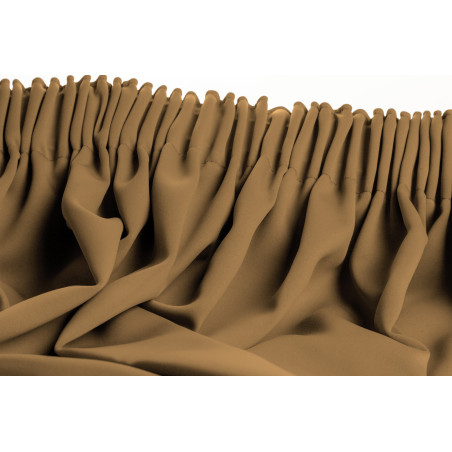
Beige BLACKOUT Curtain Solid Color Fawn MC44
FINAL SALE: This item cannot be returned.
Ideal for a bedroom or a room without shutters, this Moondream Solid Color Blackout Curtain blocks up to 100% of all incoming light* whenever you wish. It preserves your sleep and protects your privacy by isolating you from prying eyes. Woven with black threads which give it its blackout property, this Moondream curtain preserves the softness of the fabric and its elegance. Sturdy, easy to maintain and height adjustable, Moondream Cotton Effect Blackout Curtains provide a fitting last touch to your decorating schemes.
*Report N°M109 of the ENVEHO bureau dated June 2011
The curtain fabric has been produced without any harmful substances.